You may love GMAT word problems or you may hate them, but you can’t get around them if you want to ace the GMAT Quant section. No matter what your feelings are about this problem type, though, Magoosh’s experts have put together everything you need to know (and practice!) GMAT word problems in order to master them before test day.
Table of Contents
- What to Expect from GMAT Word Problems
- Strategy Guide: What’s the Trick to Mastering GMAT Word Problems?
- GMAT Word Problem Practice Questions
- A Final Word on GMAT Word Problems
What to Expect from GMAT Word Problems
Think of GMAT word problems as questions that ask you to turn real-world situations into math problems. Of course, this can be a lot more complicated than it sounds. After all, it’s one thing to understand algebra in the abstract, and quite another to think about where the rubber meets the road.
Think about it this way: the reason human beings created algebra was to solve problems about real-world situations, and the GMAT loves asking math problems about numbers and about real-world situations, a.k.a. word problems! Even folks who can do algebra in the abstract sometimes find word problems challenging.
You’ll find GMAT word problems in the GMAT Quant section. How much of the GMAT is word problems? Within the Quant section, actually a whole lot! A study of official GMAT questions from actual tests show that word problems account for 58.2% of all GMAT math questions.
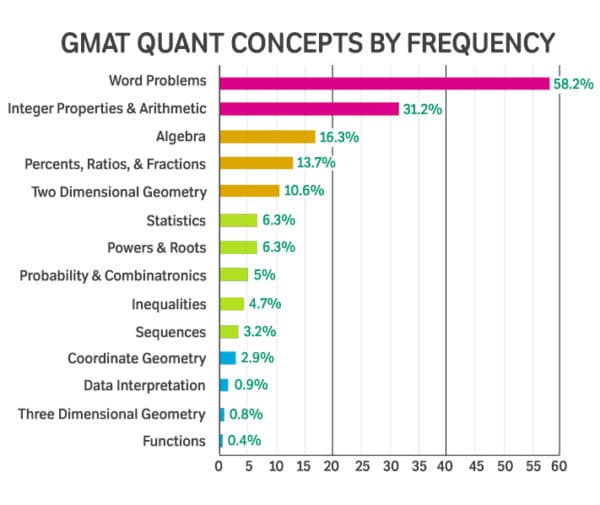
In other words, test-takers should anticipate a word problem cropping up (on average) in three out of every five questions you’ll see in Quant. Because of GMAT word problems’ prevalence, you can expect to see both Data Sufficiency and Problem Solving questions in this format. The question format and answer choices may look different, but the basic premise will be the same.
You may be feeling the pressure, but hang in there! If you’re worried about how to master word problems on the GMAT, keep reading for our GMAT Word Problems strategy guide.
Strategy Guide: What’s the Trick to Mastering GMAT Word Problems?
First, the disappointing news: there’s no one strategy that will work to immediately solve every word problem, every time (where would the fun in that be?). The good news: by using and combining a variety of strategies, you can put together the tools you need to ace even the most complex GMAT word problems, every single time!
With that in mind, here are the four key strategies you’ll need.
1. Translate from Words to Math
Suppose we have the following sentence in a word problem:”Three-fifths of x is 14 less than twice y squared.” How do we change words to math? Here’s a quick guide:
- The verb “is/are” is the equivalent of an equal sign; the equal sign in an equation is, in terms of “mathematical grammar,” the equivalent of a verb in a sentence. Every sentence has a verb and every equation has an equal sign.
- The word “of” means multiply (often used with fractions and percents). Ex. “26% of x” means (0.26x)
- The words “more than” or “greater than” mean addition. Ex. “5 greater than x” means (x + 5) and “7 more than y” means (y + 7)
- The words “less than” means subtraction. Ex. “8 less than Q” means (Q – 8). Notice that the first element is always subtracted: in other words, “J less than K” means (K – J).
With that in mind, let’s go back to the sentence from the hypothetical problem above.
- “three fifths of x” means [(3/5)*x]
- “is” marks the location of the equal sign
- “twice y squared” means 2(y^2)
- “14 less than twice y squared” means 2(y^2) – 14
Altogether, the equation we get is:
![]()
Using this strategy, it’s straightforward to translate from a verbal statement about numbers to an equation.
2. Learn to Work with Variables
Working with Variables Part I: Assigning Variables
Most GMAT word problem concern real world quantities and are stated in real world terms, and we need to assign algebraic variables to these real-world quantities.
Sometimes, one quantity is directly related to every other quantity in the problem. For example:
“Sarah spends 2/5 of her monthly salary on rent, 1/12 of her monthly salary on auto costs including gas and insurance, and 1/10 of her monthly salary automatically goes into saving each month. With what she has left each month, she spend she spends $800 on groceries and …”
In that problem, everything is related to “monthly salary,” so it would make a lot of sense to introduce just one variable for that, and express everything else in terms of that variable. Also, please don’t always use the boring choice of x for a variable! If we want a variable for salary, you might use the letter S, which will help you remember what the variable means! If we are given multiple variables that are all related to each other, it’s often helpful to assign a letter to the variable with the lowest value, and then express everything else in terms of this letter.
If there are two or more quantities that don’t depend directly on each other, then you may well have to introduce a different variable for each. Just remember that it’s mathematically problematic to litter a problem with a whole slew of different variables. You see, for each variable, you need an equation to solve it. If we want to solve for two different variables, we need two different equations (this is a common Word Problem scenario). If we want to solve for three different variables, we need three different equations (considerably less common). While the mathematical pattern continues to extend upward from there, more than three completely separate variables is almost unheard of on GMAT math.
When you assign variables, always be hyper-vigilant and over-the-top explicit about exactly what each variable means. Write a quick note to yourself on the scratch paper: T = the price of one box of tissue, or whatever the problem wants. What you want to avoid is the undesirable situation of solving for a number and not knowing what that number means in the problem!
Practice Question
Here’s a word problem practice question that’s a bit easier than what you might see on the GMAT!
Click here for a text answer and explanation
The obvious choices for variables are A = the amount in Andrew’s account and B = the amount in Beatrice’s account. The GMAT will be good about giving you word problems involving people whose names start with a different letter so that it’s easier to assign variables. We can turn the second & third sentences into equations.
second sentence: B = 3A – 600
![]()
Both equations are solved for B, so simply set them equal.
3A – 600 = 2(A + 300)
3A – 600 = 2A + 600
A – 600 = 600
A = 1200
We can plug this into either equation to find B. (BTW, if you have time, an excellent check is to plug it into both equations, and make sure the value of B you get is the same!)
B = 3000
Thus, Andrew has $1200 in his account, and Beatrice, $3000 in hers.
Working with Variables Part II: Choosing Your Approach
When questions have variables in the answer choices, you can decide whether you’d rather take an algebraic approach or a numerical approach. Neither one of these is “better” than the other— it all depends on what works best for you.
An algebraic approach is what you most likely learned back in high school. This means that, to solve the problem, you’ll manipulate the variables according to mathematical rules. For a super-basic example, to solve \( 2x = y \), you would divide both sides by two and end up with \( x = y/2 \).
However, you could also to a numerical approach to this (and many other!) problems. This means putting numbers into both the question and the answer choices. So let’s take the previous example—which, again, is much, much easier than anything you’d see on the GMAT:
If \( 2x = y \), what is x in terms of y?
A. \(y/20\)
B. \(y/2\)
C. \(4/y\)
D. \(20/y\)
Here, you could pick a number to stand in for x in the original equation. Let’s say x is 2. If x = 2, then the original equation tells us that y = 4.
Now, plug that into the question and the answer. The question becomes: “What is 2 in terms of 4?” It’s ½, or .5. Then, look for the answer choice that gives you this answer. Plugging in the numbers, you get:
A. \(y/20\) = 0.2
B. \(y/2\) = 0.5
C. \(4/y\) = 1
D. \(20/y\) = 5
So B must be correct.
What does this look like in practice? For some harder examples, take a look over at Mike’s post on Variables in GMAT Answer Choices: 2 Approaches.
3. Plug in Numbers (the Smart Way)
If you choose to use the numerical approach described above, keep in mind that there are some key tips for plugging in numbers that you should use!
Here’s a quick summary of how to quick the best numbers for a particular problem:
- Remember that the GMAT has a broad definition of “number” that goes beyond positive integers! Zero, fractions, and negatives are all included. Work on developing number sense to help select the best numbers in a given scenario.
- For percent problems, think outside the box: GMAT test writers know lots of students pick 100. Try 500 or 1000 instead.
- Don’t try to pick numbers for questions involving more than one percent increase or decrease.
- Pay attention to units and convert them appropriately. This is particularly important in solutions and mixing problems!
- Don’t pick 1 as a number—it has too many unique properties.
A separate case involving plugging in, rather than picking, numbers: When all the answer choices are numerical, one further strategy we have at our disposal is backsolving. Using this strategy, we can pick one answer, plug it into the problem, and see whether it works. If this choice is too big or too small, it guides us in what other answer choices to eliminate. Typically, we would start with answer choice (C), but if another answer choice is a particularly convenient choice, then we would start there.
4. Understand Your Strengths and Weaknesses
With all of the above strategies at your disposal, you have everything you need to improve your answers to GMAT word problems. The most efficient way to do this is to keep an error log of word problems you’ve answered wrong in your practice, then review it. As you go through, think about the following:
- What concept or concepts was the question testing?
- What was tricky about the wording of the question?
- Were you already familiar with the methods used in the explanation video?
- Once you watched the explanation video, could you explain how to solve the problem to somebody else?
Your answers to these questions can help you craft a better strategy for GMAT Math word problems, identifying exactly what you need to review to get better!
GMAT Word Problem Practice Questions
Now that you’ve learned how to approach word problems, we’ve put together a collection of them, direct from Magoosh’s product, for you to try! Video and text answers and explanations follow each question.
- Ann and Bob planted trees on Friday. What is the ratio of the number of trees that Bob planted to the number of trees that Ann planted?
(1) Ann planted 20 trees more than Bob planted.
(2) Ann planted 10 percent more trees than Bob planted.
Click here for a video answer and explanation to GMAT Word Problem 1!
Click here for a text answer and explanation to GMAT Word Problem 1!
Our task is to determine the ratio of Bob’s trees to Ann’s trees. Let’s label these numbers of trees with variables:
Bob’s trees→B, Ann’s trees→A
With these variables, we can express the ratio we want to determine:
\(B/A\) =?
Statement 1:
Ann planted 20 trees more than Bob planted.
Let’s translate this into an equation using A and B:
\( A=B+20 \)
Now we can substitute this into our ratio, replacing A:
\(B/A\) = \( B/(B+20) \)
No matter what simplifications we make, we cannot find a numerical value for this fraction. We would need a value for B. We cannot determine the ratio. Statement 1 by itself is not sufficient.
Statement 2:
Ann planted 10 percent more trees than Bob planted.
Let’s translate this into an equation using A and B:
\(A=1.10 x B \)
Again, let’s substitute this in for A in our ratio:
\(B/A\) = \( B/(1.10B) \)
= \(1/1.1 \)
We found a value for the ratio of Bob’s trees to Ann’s trees. Statement 2 alone is sufficient.
- The Townville museum was open for 7 consecutive days. If the number of visitors each day was 3 greater than the previous day, how many visitors were there on the first day?
(1) There were a total of 126 visitors for the 7 days.
(2) The number of visitors on the seventh day was three times the number of visitors on the first day.
Click here for a video answer and explanation to GMAT Word Problem 2!
Click here for a text answer and explanation to GMAT Word Problem 2!
If x is the number of visitors on the first day, then:
x = # of visitors on the 1st day
x + 3 = # of visitors on the 2nd day
x + 6 = # of visitors on the 3rd day
x + 9 = # of visitors on the 4th day
x + 12 = # of visitors on the 5th day
x + 15 = # of visitors on the 6th day
x + 18 = # of visitors on the 7th day
1) Adding up the number of visitors gives us:
x + (x + 3) + (x + 9) + (x + 12) + (x + 15) + (x + 18) = 126
We could simplify and solve this for x. So Statement 1 is sufficient.
2) x + 18 = 3x
Again, we can simplify this and solve for x. So Statement 2 is sufficient.
Answer: (D)
- Two teachers, Ms. Ames and Mr. Betancourt, each had N cookies. Ms. Ames was able to give the same number of cookies to each one of her 24 students, with none left over. Mr. Betancourt was also able to give the same number of cookies to each one of his 18 students, with none left over. If N > 0, what is the value of N?
(1) N<100
(2) N > 50
Click here for a video answer and explanation to GMAT Word Problem 3!
Click here for a text answer and explanation to GMAT Word Problem 3!
This question is really about common multiples and the LCM (note that it is different than finding the set of all multiples, though!). If Ms. Ames can give each of her 24 students k cookies, so that they all get the same and none are left over, then 24k = N. Similarly, in Mr. Betancourt’s class, 18s = N.
What are the common multiples of 18 and 24?
18 = 2×9 = 2×3×3 = 6×3
24 = 3×8 = 2×2×2×3 = 6×4
From the prime factorizations, we see that GCF = 6, so the LCM is
LCM = 6×3×4 = 72
and all other common multiples of 18 and 24 are the multiples of 72: {72, 144, 216, 288, 360, …}
Statement #1: if N<100, the only possibility is N = 72. This statement, alone and by itself, is sufficient.
Statement #2: if N > 50, then N could be 72, or 144, or 216, or etc. Many possibilities. This statement, alone and by itself, is not sufficient.
Answer = (A)
- A certain zoo has mammals and reptiles and birds, and no other animals. The ratio of mammals to reptiles to birds is 11:8:5. How many birds are in the zoo?
(1) there are twelve more mammals in the zoo than there are reptiles
(2) if the zoo acquired 16 more mammals, the ratio of mammals to birds would be 3:1
Click here for a video answer and explanation to GMAT Word Problem 4!
Click here for a text answer and explanation to GMAT Word Problem 4!
A short way to do this problem. The prompt gives us ratio information. Each statement gives use some kind of count information, so each must be sufficient on its own. From that alone, we can conclude: answer = D. This is all we have to do for Data Sufficiency.
Here are the details, if you would like to see them.
Statement (1): there are twelve more mammals in the zoo than there are reptiles
From the ratio in the prompt, we know mammals are 11 “parts” and reptiles are 8 “parts”, so mammals have three more “parts” than do reptiles. If this difference of three “parts” consists of 12 mammals, that must mean there are four animals in each “part.” We have five bird “parts”, and if each counts as four animals, that’s 5*4 = 20 birds. This statement, alone and by itself, is sufficient.
Statement (2): if the zoo acquired 16 more mammals, the ratio of mammals to birds would be 3:1
Let’s say there are x animals in a “part”—this means there are currently 11x mammals and 5x birds. Suppose we add 16 mammals. Then the ratio of (11x + 16) mammals to 5x birds is 3:1.
(11x + 16)/(5x) = 3/1 = 3
11x + 16 = 3*(5x) = 15x
16 = 15x – 11x
16 = 4x
4 = x
So there are four animals in a “part”. The birds have five parts, 5x, so that’s 20 birds. This statement, alone and by itself, is sufficient.
Both statements are sufficient. Answer = D.
A Final Word on Word Problems
So, what is the trick to GMAT word problems? As you’ve seen in this post, there’s no one-size-fits-all trick—but there are plenty of strategies!
The strategies you’ve read about here can be used to take the given information and identify key words in a question. With them, you’ll be able to find everything from average speed to total distance traveled, from total time to total amount.
The key now is to put them into practice. Jot down these techniques or bookmark this post so you can come back as you continue your practice with GMAT word problems. You can also check out our posts on compound interest and Venn diagrams for more practice with GMAT word problems. Good luck!
This post was written with contributions from our Magoosh content creator, Rachel Kapelke-Dale.





Leave a Reply