In the first three articles in this series on GMAT probability questions, I discussed the AND and OR probability rules, “at least” probability questions, and probability questions that involve counting. This post covers relatively rare kind of probability question not covered in the first three posts: geometric probability questions. First, a few challenging practice GMAT questions on this topic.
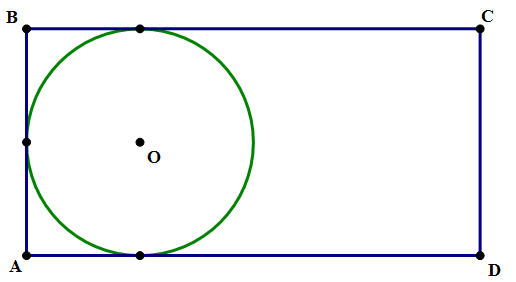
1) In the diagram above, the sides of rectangle ABCD have a ratio AB:BC = 1:2, and the circle is tangent to three sides of the rectangle. If a point is chosen at random inside the rectangle, what is the probability that it is not inside the circle?
2) Region R is a square in the x-y plane with vertices J = (–1, –2), K = (–1, 4), L = (5, 4), and M = (5, –2). What is the probability that a randomly selected point in region R lies below the line 3x – 5y = 10?
-
(A) 5/12
(B) 5/18
(C) 5/24
(D) 5/36
(E) 5/72
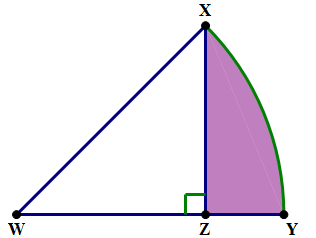
3) In the diagram above, WZ = XZ, and circular arc XY has a center at W. If a point is selected from anywhere within this figure, what is the probability that it is selected from the shaded region?
These three are very challenging problems. If they seem easy to you, you are probably a GMAT Quantitative expert! If these make your head spin, please read on!
Probability and area
As anti-intuitive as this may seem, there is a deep mathematical link between probability and area. For example, if you are dealing with regions under the Normal Distribution or any other distribution, the probability of landing in those regions is measured by finding the area under the curve. This connection between area and probability because quite apparent if you happen to study calculus-based statistics. If you haven’t ventured into such regions, I’ll just say, remember: there’s an important link between probability and area. In particular, if a diagram is given or described in a GMAT Quantitative question, and you are asked for a probability, then you almost always will be finding a ratio of two areas. Thus, any geometric probability problem just becomes a 2-in1 find-the-area problem.
Finding area
Know your basic geometry. You should know how to find the area of rectangles and triangles: if you don’t remember, say, the formula for the area of a trapezoid, just break it into triangles & rectangles and find the area piece-by-piece. You should know Archimedes‘ remarkable formula for the area of a circle:
You should also be comfortable with setting up the proportion necessary to find the area of a circular sector (i.e. a “slice of the pie”).
Visualize
If you are given a diagram as part of the problem, that’s great. If you are not given a diagram, sketch one on the notepad. In any GMAT problem involving Geometry, it’s very important to have your right-brain visual skills engaged.
A ratio of areas
The most general formula for probability is
In the case of geometry probability, each of these terms, the numerator and the denominator, is an area.
Many times in geometric probability problems, as in #1 and #3 above, no absolute lengths are given — we know the relative ratios, but we don’t know in absolute terms how big anything is. The information in the problem does not allow us to compute the numerical value of any area. That’s fine. Just pick any convenient number for one of the lengths, and figure out everything from there. It doesn’t matter what value you pick, because in the probability ratio, the value you pick will cancel. All that matters are the ratios.
Summary
With these recommendations, go back and look at those problems again before reading the explanations below. Here’s another practice question from inside the product:
4) http://gmat.magoosh.com/questions/829
Practice question explanations
1) We aren’t given any absolute lengths. For convenience, I am going to assume that the radius of the circle is r = 1. That’s very easy. Right away, we know the area of the circle is <m>pi</m>. Notice, the height of the triangle is equal to the diameter of the circle, so h = AB = 2. We are told the ratio of AB:BC = 1:2, so BC, the width, must equal w = BC = 4. Area of the rectangle is h*w = (AB)*(BC) = 8. That, right there, is our “denominator area”. Now, for the numerator area, the area of the rectangle that does not include the circle, subtract the circle from the rectangle: A = <m>8 – pi</m>. That’s our numerator.
Answer = D
2) Here, we are not given a diagram, so we will sketch one. In the real test, just a rough diagram will be enough to visualize things. Here’s an accurate diagram.
Notice the square JKLM has an area of 6 x 6 = 36 —- right there, that’s our denominator-area.
Now, let’s think about this line. Let’s solve for y, to put the line into slope-intercept form (i.e. y = mx + b form).
First of all, putting it in slope-intercept form makes clear — the y-intercept of this line is point S, (0, –2), which is on one side of the square. The slope is 3/5, so over five and up three puts us at point T, (5, 1), also on a side of the square. Triangle STM has a base of 5 and height of three, so the area is
That’s the numerator-area, so just divide
Answer = C
3) Again, we are not given any absolute lengths, so we will pick something convenient. Here, I am going to pick WZ = XZ = 1. Triangle WXZ is an Isosceles Right Triangle, i.e. a 45-45-90 triangle. Its area is A= (1/2)bh, and since both base and height are 1, the area of the triangle is 1/2.
Both WX and WY are radii of the circle. From the proportions in the 45-45-90 triangle, we know
Now, we can find the area of the circle, using Archimedes’ formula:
Because triangle WXZ is a 45-45-90 triangle, we know ∠W = 45°. Well, a 45° angle must be half of 90° —- which means a 45° is 1/4 of 180° —- which means a 45° is 1/8 of 360°. Thus, a 45° sector would be 1/8 of a circle and have 1/8 of the entire circle’s area.
First of all, that’s the denominator-area in our probability. Also, we can use this to figure out the area of the shaded region.
Area of shaded region = (area of sector) – (area of triangle)
That’s our numerator-area. Now, we can put together the probability — we will just have to simply the complex fraction by multiplying numerator and denominator by 4.
Answer = A
Try your hand at a few more non-geometric GMAT Probability questions.

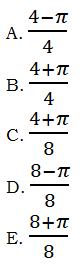
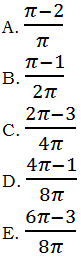

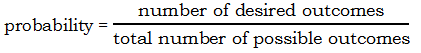
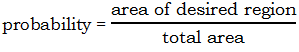


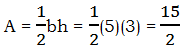

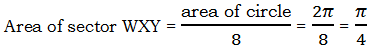
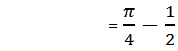





Leave a Reply