Permutations
A permutation is a possible order in which to put a set of objects. Suppose I had a shelf of 5 different books, and I wanted to know: in how many different orders can I put these 5 books? Another way to say that is: 5 books have how many different permutations?
In order to answer this question, we need an odd math symbol: the factorial. It’s written as an exclamation sign, and it means: the product of that number and all the positive integers below it, down to 1. For example, 4! (read “four factorial”) is
4! = (4)(3)(2)(1) = 24
Here’s the permutation formula:
# of permutations of n objects = n!
So, five books 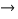 the number of permutations is 5! = (5)(4)(3)(2)(1) = 120
the number of permutations is 5! = (5)(4)(3)(2)(1) = 120
Combinations
A combination is a selection from a larger set. Suppose there is a class of 20, and we are going to pick a team of three people at random, and we want to know: how many different possible three-person teams could we pick? Another way to say that is: how many different combinations of 3 can be taken from a set of 20?
This formula is scary looking, but really not bad at all. If n is the size of the larger collection, and r is the number of elements that will be selected, then the number of combinations is given by
# of combinations = 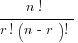
Again, this looks complicated, but it gets simple very fast. In the question just posed, n = 20, r = 3, and n – r = 17. Therefore,
# of combinations = 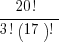
To simplify this, consider that:
20! = (20)(19)(18)(17)(the product of all the numbers less than 17)
Or, in other words,
20! = (20)(19)(18)(17!)
That neat little trick allow us to enormously simplify the combinations formula:
# of combinations = 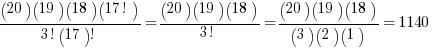
That example is most likely harder than anything you’ll see on the GMAT math, but you may be asked to find combinations with smaller numbers.
Practice Questions
1) A bookseller has two display windows. She plans to display 4 new fiction books in the left window, and 3 new non-fiction books in the right window. Assuming she can put the four fiction books in any order, and separately, the three non-fiction books in any order, how many total configurations will there be for the two display windows?
-
(A) 24
(B) 72
(C) 144
(D) 336
(E) 420
2) The county-mandated guidelines at a certain community college specify that for the introductory English class, the professor may choose one of three specified novels, and choose two from a list of 5 specified plays. Thus, the reading list for this introductory class is guaranteed to have one novel and two plays. How many different reading lists could a professor create within these parameters?
-
(A) 15
(B) 30
(C) 90
(D) 150
(E) 360
Answers and Explanations
1) The left window will have permutations of the 4 fiction books, so the number of possibilities for that window is
permutations = 4! = (4)(3)(2)(1) = 24
The right window will have permutations of the 3 non-fiction books, so the number of possibilities for that window is
permutations = 3! = (3)(2)(1) = 6
Any of the 24 displays of the left window could be combined with any of the 6 displays of the right window, so the total number of configurations is 24*6 = 144
Answer: C.
2) There are three possibilities for the novel. With the plays, we are taken a combination of 2 from a set of 5 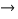 n = 5, r = 2, n – r = 3
n = 5, r = 2, n – r = 3
# of combinations = 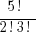 =
= 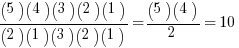
If the plays are P, Q, R, S, and T, then the 10 sets of two are PQ, PR, PS, PT, QR, QS, QT, RS, RT, & ST.
Any of the three novels can be grouped with any of the 10 possible pairs of plays, for a total of 30 possible reading lists.
Answer: B.
Special Note:
To find out where permutations and combinations sit in the “big picture” of GMAT Quant, and what other Quant concepts you should study, check out our post entitled:
What Kind of Math is on the GMAT? Breakdown of Quant Concepts by Frequency





Leave a Reply