For some this is the most “interest”ing concept on the Revised GRE (at least for those with a fondness for bad puns). For most, compound vs simple section can be a nuisance. Many think, what is the difference between the two, and/or how did that formula go again?
But remember, this concept involves money, and for many that means its practical (especially if you invest money yourself). But enough rambling…
Simple Interest
Principal: The Amount of Money initially invested
Interest Rate: The amount return on an investment expressed as a percent of the principal.
Time: The length of time in which a principal is invested
Sample Problems
1. John invests 100 dollars in account that yields 8% simple interest annually. How much money will John have in his account after one year?
(A) $4
(B) $8
(C) $104
(D) $108
(E) $110
2. Bob invests 100 dollars in a fund that yields 15% simple interest monthly. If Bob invests the principal in the middle of January, which is the first month will he have more than $200 total?
(A) June
(B) July
(C) August
(D) February
(E) March
3. In 2001, John invests x dollars in a special account that yields y% simple interest annually. If he has $250 in his account in 2006 and in 2008 he has $270 in his account, what is x + y?
(A) 5
(B) 25
(C) 200
(D) 205
(E) 210
Answers:
1. D
2. C
3. D
Compound Interest
Okay, that was the easy part. Now for compound interest. In compound interest things become complicated. We no longer have a nice, clean linear increase. To illustrate:
If Mike invests $100 at 10% simple annual interest, he will have $110. After two years he will have $120. That is his money grows by $10 every year. After 10 years, Mike will have doubled his money.
Now, let’s say Mike’s friend Thomas invests $100 at a 10% rate that is compounded annually. After one year, Thomas will have made the same amount as Mike. But then things start diverging. Remember how Mike always gets 10% of the original 100 (the original 100 is called the principal)? Well, Thomas – because things are getting compounded annually – gets 10% of whatever the value of the account is at the end of the year. Let’s see how this plays out over time.
1st year: 10% of 100 = 110
2nd year: 10% of 110 = 121
3rd year: 10% of 121 = 133.10
4th year: 10% of 133.00 (rounding down) = 146.30…
After 10 years, Thomas will have made $260, which is $60 more than Mike.
Okay, that may all seem like chump change, but the same percent increase applies to numbers with a few more zeroes thrown in. How would $260,000 vs. 160,000 sound?
Of course the point of this lesson is to understand the conceptual difference between the two forms of interest—and not to have you running to the nearest ban, since the numbers above are very generous.
Now for the fun part: Notice how, in the case of Thomas, I seemed to be doing mathematical wizardry. After all, how did I know that 10% compounded annually at 10 years is going to yield 160% of the principal? Well, let’s meet the formula:
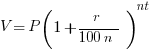
V = Total Value
P = Principal
r = annual interest rate
n = number of times per year invested
t = number of years
Pretty unpleasant, no? Well, let’s try to put the formula to the test. And you may want to get your calculators out (this is the Revised GRE after all!)
If $10,000 is invested at 10%, compounded semi-annually, how much will the investment be worth after 18 months?
(A) 11,500
(B) 11,505
(C) 11,576.25
(D) 11,625.30
(E) 12,000.50
Now don’t worry about the semi-annual bit—it just means twice a year. And remember the n from the scary little formula above: the number of times per year invested. And that 18 months? That corresponds to t, the number of years, which translates to 1.5.
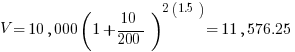 .
.
That was easy—once you know where to put everything (and provided you remember the formula)!




Leave a Reply