The AP Calculus AB covers the equivalent material of a single semester of calculus at the college level. In fact, this test covers a bit more than that — roughly one and a half semesters of calculus. This article outlines the topics that you may expect to see on the AP Calculus AB exam.
Topics on the AP Calculus AB Exam
The material on the exam falls into three main categories, limits, derivatives, and integrals. However these topics are deeply intertwined. It’s not uncommon to encounter a free response question that requires methods from all three areas.
Limits and Continuity

Limits have to do with how graphs of functions behave. As x approaches a number a, what happens to the values of f(x)? Related topics include asymptotes of graphs and theorems that require continuity.
- Graphing and interpreting graphs (pre-calculus).
- Limits and continuity. Finding limits algebraically or estimating them from numerical or graphical data. Continuity in terms of limits.
- Intermediate Value Theorem and Extreme Value Theorem.
- Vertical, horizontal, and oblique asymptotes. Limits involving infinity.
Derivatives and Rates of Change
The derivative measures the slope of the graph at a point. However, derivatives also measure rate of change (such as velocity), and can be used to find out where functions increase and decrease. Be aware that there are various rules and formulas for finding derivatives.
- Limit definition of the derivative and its relationship to continuity.
- Derivative rules including the Power Rule, Product Rule, Quotient Rule, and Chain Rule.
- Slope and tangent lines.
- Linear approximation and differentials.
- Instantaneous and average rates of change. Relationship among position, velocity, and acceleration functions.
- Higher order derivatives.
- Implicit Differentiation.
- Analysis of Graphs based on both pre-calculus methods and derivative information. This includes finding intervals of increase/decrease, relative minima/maxima, intervals of concavity, and inflection points.
- Mean value theorem and Rolle’s Theorem.
- Applications of derivatives, including optimization and related rates.
- Elementary differential equations and slope fields.
Integrals and Area
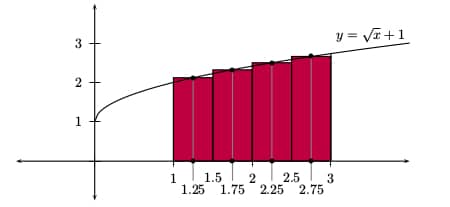
Integrals cover many related ideas, including antidifferentiation and the Fundamental Theorem of Calculus. They can be used to compute areas and volumes. Additionally, integrals play an important role in applied problems in mathematics.
- Antidifferentiation and indefinite integrals.
- Techniques of antidifferentiation, including power rule, algebraic manipulation, and substitution.
- Finite Riemann sums and their limits. Relationship to definite integrals.
- The Fundamental Theorem of Calculus and definite integrals.
- Trapezoid Rule and other methods for estimating area.
- Exact area below a curve or between two curves, using definite integrals.
- Volumes of solids of revolution, by washer method and shell method.
- Accumulation functions.
- Relationships between position, velocity, and acceleration using integrals.
- Average value of a function over an interval.
- Models for exponential growth and decay.
Final Thoughts
If you score a 4 or 5 on the AP calculus AB exam, then most colleges and universities will offer 4-5 credits of calculus (single semester). Sometimes even a score of 3 will earn you credit for lower level courses such as Precalculus. However, schools differ in their AP policies, so always check out that information before applying.
More detailed descriptions of the topics covered on both the AB and BC test can be found here.





Leave a Reply