The Revised GRE is a long, grueling test. To get a perfect score on the quantitative section (a 170) you will not only have to answer the difficult questions correctly, but you will also have to answer all of the easy ones. Nonetheless, I’ve focused on the difficult questions for the 170-challenge. I can’t promise a perfect correlation, but something tells me that if you can answer all five of these questions correctly in less than 10 minutes, you are well on your way to a perfect score.
Of course missing a few hardly precludes a perfect score. But make sure you learn from the ones you miss to make sure you don’t make similar mistakes in the future. And if time is a problem, remember the Magoosh GRE product has plently of questions to help you hone your chops—both from a pacing and a conceptual standpoint.
Good luck, and feel free to post your answers. Let’s see who the first perfect score will be!
1. 
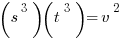If s and t are both primes, how many positive divisors of v are greater than 1, if v is an integer?
(A) two
(B) three
(C) five
(D) six
(E) eight
2. A quadrilateral has a perimeter of 16. Which of the following alone would provide sufficient information to determine the area of the quadrilateral. Choose ALL that apply.
[A] The quadrilateral contains equal sides
[B] The quadrilateral is formed by combining two isosceles right triangles
[C] Two pairs of congruent angles are in a 2:1 ratio
[D] The width is 4o% of the length and all angles are of equal measure
[E] If the perimeter was decreased by 50%, the area would decrease to 25% of the original
3. Product Question: Triangle in a Parabola
http://gre.magoosh.com/questions/2192
4. If x is an integer, and 169 <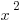 
< 324, which of the following is the sum of all values of x?
(A) 61
(B) 62
(C) 75
(D) 93
(E) None of the above.
5. x = 350,000
y = 45,000
| Column A | Column B |
|---|---|
| The total number of positive divisors of x | The total number of positive divisors of y |
- The quantity in Column A is greater
- The quantity in Column B is greater
- The two quantities are equal
- The relationship cannot be determined from the information given
[Note from our intern, Dylan: “I can do multivariable calculus, and got 2/5 on these in 10 minutes. These guys are tricky. :P”]
Explanation Video #1
https://www.youtube.com/watch?v=BxkB8R7esB4
Explanation Video #2
https://www.youtube.com/watch?v=jZdxqajkZtw
Explanation Video #3
http://gre.magoosh.com/questions/2192
Explanation Video #4
https://www.youtube.com/watch?v=pwzlclrvqWU
Explanation Video #5
https://www.youtube.com/watch?v=–U8aDmLNzE
Most Popular Resources
