We recently issued a call for you, and other students, to submit questions that we could answer in a video series. There were a lot of great submissions, and the ones that we thought useful for a broad swath of students, we answered in our video series. These videos will be released over the next few weeks.
But some of the questions require a longer explanation. So in this post, I want to answer those other questions that came in.
Question 1
Of the 120 passengers on Flight 750, 50% are female. 10% of the passengers sit in first class, and the rest of the passengers sit in coach class. If 1/3 of the passengers in first class are male, how many females are there in coach class?
44
48
50
52
56
Here we have a math problem that you might see on the GMAT. This question would rank as an easy to medium level question on the test. Let’s look at how to solve this problem.
First thing I always do when I approach a problem is wade through the information and write down the facts. So, here is what I’m told:
- 120 people
- 50% female
- 10% of passengers sit in first class
- ⅓ of passengers in first class are male
Now, I will interpret this information and see what else it tells me:
- 50% female means there are 60 males and 60 females on the plane
- 10% of passengers sit in first class means that 12 people are seated in first class. If 12 people are in first class, there are 108 people in coach.
- ⅓ of passengers in first class are male, which means that 4 males and 8 females are seated in first class
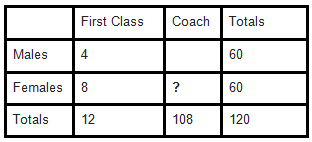
Now our job is to find out how many females are seated in coach. This may seem like a tall order, but if we use a Double Matrix this will be fairly simple. First we need to make a table with what we know:
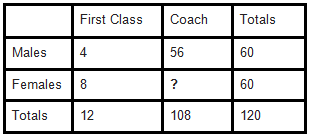
Alright now we can start filling in our boxes with the other information and we will be able to solve for how many females are sitting in coach.
Now with some simple subtraction (108 – 56 = 52 or 60 – 8 = 52), we have our answer. You could’ve used a Venn diagram to find the solution, but I find the Double Matrix more straightforward. If you don’t, spend some time learning about the Double Matrix because it is an important tool have in your math toolbox.
Question 2
What is a ratio?
This is a question that we have addressed in a few other posts (here, here, and here). And I am not sure if I can provide a better explanation than some of these other resources (Wikipedia or Khan Academy), but I will give it a shot.
Simply stated, a ratio expresses a relationship between two things. These two things are connected for some reason and exert some influence on each other. Whether it is the relationship between miles and time, the relationship between a distance on a map to an actual distance, or the relationship of teachers to students in a school, they all tell us something about the relationship.
For example, when we talk about the speed limit, we are talking about a relationship, one of the fundamental relationships in the universe—time and distance. So speed is really just a ratio that tells us how far we have traveled, or could travel, in a certain amount of time.
A ratio can be expressed two different ways—with a colon (:) or with a fraction bar (/). As we know with speed, we usually say “per” to indicate the fraction bar or the colon. So when your friend turns to you and says, “We’re going a 165 miles per hour,” you could write this as:
165 miles : 1 hour
165 miles / 1 hour
One crucial point to know is that a ratio doesn’t necessarily tell you totals. Let me show you what I mean. If I told you that a school has 1 teacher for every 32 students, I haven’t told you anything that helps you know how many students are at the school and how many teachers are at the school.
But with another piece of information, you’d be able to figure out all this information. So, if I told you that there are 12 teachers at the school, then we can determine how many students are at the school. If 1 teacher has 32 students, then 2 teachers have 64 students, and 3 teachers have 96 students, and…. Hopefully, you can see that we are just counting by 32 until we have counted 12 teachers. That is the same as a simple multiplication formula: 12 * 32 = 384.
So this is not the end all for your understanding of ratios—this is the beginning! Take the time to review some of the other resources to deepen your understanding of ratios.






Leave a Reply