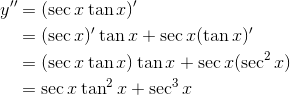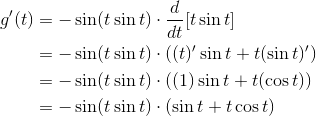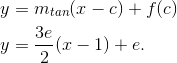AP Calculus Review: Product Rule

By

Shaun Ault

on

in

The product rule is just one of many essential derivative rules. Together with the Sum/Difference Rule, Power Rule, Quotient Rule, and Chain Rule, these rules form the backbone of our methods for finding derivatives. In this article I’ll explain what the Product Rule is and how to use it in typical problems on the AP Calculus exams.

The Product Rule

Suppose f and g are differentiable functions. Then the product function, fg is also differentiable, and

Product Rule

Here is another way to state the rule in a more compact way:

Product Rule written in terms of u and v

Many textbooks write the terms in different orders. Here is yet another version of the rule, this time using Leibniz notation.

Product Rule in Leibniz notation

Example 1

Find the derivative of f(x) = x5 sin x.

This function is the product of two functions, u = x5 and v = sin x. Using the Product Rule,

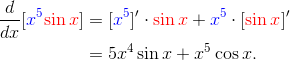
Using the product rule. Example 1.

Note, the correct derivative is not found by just taking the product of the derivatives. In other words, f '(x) ≠ 5x4 cos x.

Example 2

In the next example, we’ll see that the Product Rule can even be used when very little information is known about the individual functions.

Suppose f and g are differentiable at x = 4, and suppose we know that f(4) = -3, g(4) = 2, f '(4) = 7, and g '(4) = 3. Let h = fg. What is the value of h '(4)?

Be careful! The answer is not 21. Instead, set up the rule and then plug in the known information:

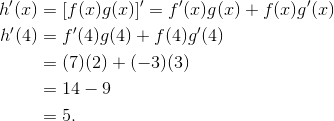
Product Rule example 2, worked out.

Practice Problems

Now’s your chance to practice using the Product Rule! Solutions are provided at the end.

  1. Find the derivative of Practice Problem 1.
  2. If y = sec x, what is y ''?
  3. (Requires Chain Rule) Differentiate g(t) = cos(t sin t).
  4. Find the equation of the tangent line to the curve Practice Problem 4 at x = 1.

Thinking cap -Magoosh

Solutions

  1. Solutions to Practice Problem 1
  2. The first derivative is: y ' = sec x tan x. The Product Rule is required to find the second derivative:

    Solution to Practice Problem 2

  3. Use the Chain Rule first, and then Product Rule for the derivative of the “inside” function.
    Solution for Practice Problem 3
  4. To find the equation of the tangent line, we will need the slope. And that requires finding a derivative.

    Solution to Practice Problem 4, part A

    Plug in x = 1 to find the slope.

    Finding the slope.

    Finally, we need the y-coordinate of the point. Plug x = 1 back into the original function to get: y = e. Now we can put together the tangent line equation:

    Solution to Practice Problem 4 - tangent line equation

Author

  • Shaun Ault

    Shaun earned his Ph. D. in mathematics from The Ohio State University in 2008 (Go Bucks!!). He received his BA in Mathematics with a minor in computer science from Oberlin College in 2002. In addition, Shaun earned a B. Mus. from the Oberlin Conservatory in the same year, with a major in music composition. Shaun still loves music — almost as much as math! — and he (thinks he) can play piano, guitar, and bass. Shaun has taught and tutored students in mathematics for about a decade, and hopes his experience can help you to succeed!

    View all posts

More from Magoosh

Share
Tweet
Pin