GMAT OG Case Study: Problem Solving Practice Problem #163

Here is the problem that appears in the OG, 12th Edition:

Problem Solving Practice Problem #163

This year Henry will save a certain amount of his income, and he will spend the rest.  Next year Henry will have no income, but for each dollar he saves this year, he will have 1 + r dollars available to spend next year.  In terms of r, what fraction of his income should Henry save this year so that next year the amount he has to spend will be equal to half the amount he spends this year?

(A)
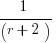
(B)
(C)
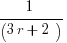
(D)
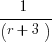
(E)

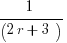
This is a doozy of a question!  It involves a complicated verbal scenario, thinking about proportions, and all the answer choices are in terms of variables.  Holy schnikies!

Notice that r is something like interest on an investment, and here, r is a decimal between 0 and 1.  We know that because we got the multiplier (1 + r) simply by adding one to r.  If r were, say, a percent between 0 and 100, we would have to divide r by 100 before adding one when we constructed the multiplier.

I will show two completely different methods to solve this: the first, a more traditional algebraic approach, and the second, a numerical approach.

Method #1: Algebraic Solution

Let’s say that x is what the question is asking — x is the proportion of income that Henry saves this year.  We will assume that x is a number between 0 and 1.

We will also use the variable T for the dollar amount of the total income that Henry makes this year.  We expect this variable to cancel out at some point.  Given these two variables, what do we know?

Well, the dollar that Henry saves

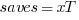
Therefore, the dollar amount he spends this year is:

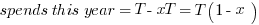
If he saves xT this year, that means the dollar amount he will have available to spend next year is:

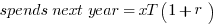
Well, the condition imposed by the last sentence in the question is:

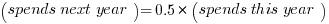
Plug in algebraic expressions into this word equation:

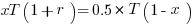
Multiply by 2 to clear the fractions/decimals:

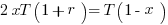
As promised, the T cancels.  Divide by T.

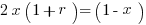
Now, we need to solve for x.  Distribute on the left side.

Add x to both sides, so that all the x’s are on the left side of the equation:

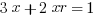
Now, the death-defying Algebra One move: factor out a factor of x:

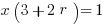
To complete the process of isolating x, divide by the parentheses:

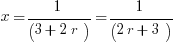
This expression is equal to Answer Choice E, so this is the correct answer.

Method Two: Plugging in Clever Numbers

Let’s pick some ridiculous easy answers, and see if we can eliminate our way to the answer.

First of all, let’s consider the extreme case where r = 0.  Henry gets no interest at all: he might as well have buried the saved portion in an old shoe under the back porch.  What he has to spend is exactly equal to what he saved.

Well, let x bethe fraction of his income that he savesnow & spends next year; then (1 – x) is the fraction he spends now.  Givenwhat he saves & spends next year is 1/2 of what he spends this year, we have:

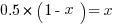
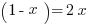
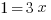
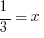
Therefore, he must spend 2/3 of his income and save 1/3 of his income.  Thus, when r = 0, the ratio Henry has to save is 1/3.

Well, if we plug zero into the answer choices, how many of them equal 1/3?  (A) & (B) & (C) do not, so we can immediately eliminate them.  One easy choice, and three potential answers are gone, leaving only (D) & (E) as viable options.

Now, an equally extreme answer: assume that r = 1.  Whatever Henry saves is doubled in one year (a pretty sweet deal!  I’d love to find that bank!)  Let x equal the fraction of his income that he saves this year.  This means he spends (1 – x) this year.  It also means, his savings doubles, so next year he spends 2x.  Again, what he spends next year is 1/2 of what he spends this year

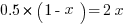
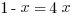
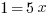
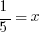
Therefore, he must save 1/5 of his income and spend 4/5 of it.  If r = 1, the ratio Henry should save is 1/5.

Plug r = 1 into remaining choices (D) & (E).  (D) doesn’t work.  (E) works.  Bingo.  We’re done, and we know the answer must be (E).