Many common questions asked on the AP Calculus Exams involve finding the equation of a line tangent to a curve at a point. If we are adept at quickly taking derivatives of functions, then 90 percent of the work for these types of problems is done. Everything else comes down to quick algebra.
The first thing we need to do is to go back to what we learned in our algebra: the equation of a line or y = mx+b, where m is our slope and b is our y-intercept. This should be at our fingertips. Now, we don’t always have our y-intercept, so a slightly different form of our equation of a line is often useful: y-y1 = m(x-x1), where m is our slope, and x1 and y1 are the coordinates of a point. Questions involving finding the equation of a line tangent to a point then come down to two parts: finding the slope, and finding a point on the line.
Let us take an example
Find the equations of a line tangent to y = x3-2x2+x-3 at the point x=1.
Firstly, what is the slope of this line going to be? Anytime we are asked about slope, immediately find the derivative of the function. We should get y’ = 3x2 – 4x + 1. Evaluate this derivative at x = 1, and we get 3(1)2 -4(1) +1 = 3-4+1= 0. The slope, m, of this function at x=1 is 0. m=0. (Note, for the AP exam, you should also be able to use the derivative of this function in a similar way to find local minimums and maximums – we should be able to see that because our slope is 0, we are looking at a line that exists at a local minimum or maximum).
Second, let us find a set of points (x1, y1) that exist on the line. At this point, we can only use one value of x, and that is the value given, x=1. To find the value y, we plug it into our original equation: y = (1)3-2(1)2+1-3 = 1-2+1-3 = -3. Therefore (x1, y1) = (1, -3). We now have both a point on our line and the slope of our line. This is everything we need to find our equation.
The equation of our line:
y-y1 = m(x-x1)
y-(-3) = 0(x-1)
y +3 = 0
y = -3
Here we have the equation with the tangent line drawn in:
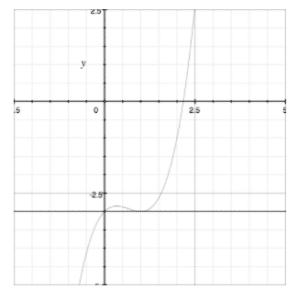
(Can you find a local maximum of this function?)
Another tangent line equation example
Let’s do the exact same question as above, but at a new point: Find the equations of a line tangent to y = x3-2x2+x-3 at the point x=2.
Again, what is the slope of this line going to be? First, the derivative: y’ = 3x2 – 4x + 1. Evaluate at x = 2.
3(2)2-4(2) +1 = 12-8+1 = 5. The slope, m, of this function at x=2 is 5 (m=5).
Set of points (x1, y1). We can only use x=2. Plug it into our original equation.
y = 23-2(2)2+2-3 = 8-8+2-3 = -1.
(x1, y1) = (2, -1).
The equation of our line:
y-y1 = m(x-x1)
y-(-1) = 5(x-2)
y +1 = 5x-10
y = 5x-11

Finding the Slope of a Tangent Line: A Review
Finding the equation of a line tangent to a curve at a point always comes down to the following three steps:
- Find the derivative and use it to determine our slope m at the point given
- Determine the y value of the function at the x value we are given.
- Plug what we’ve found into the equation of a line.
Master these steps, and we will be able to find the tangent line to any curve at any point.





Leave a Reply