
Trigonometry, or trig for short, is usually taught around 11th grade, so depending on how early you take the SAT, you may not have even learned it in your math class yet. The SAT trig is lumped into the ‘Additional Topics’ math category. If you want to be able to handle SAT trigonometry problems with ease, this guide will boil it down to what you need to remember. It’s short enough to be a refresher, yet thorough enough for the beginner.
What is Trigonometry?
To put it simply, trigonometry is a branch of math that deals with the relationship between angles and lengths of triangles. You can use it to solve for missing angle measures, side lengths, and much more once you go beyond the basic foundations.
Don’t worry if it all sounds really difficult and scary. By the end of this guide, you’ll learn the skills and know-how to crush all the SAT Trigonometry you might run into.
SAT Trigonometry: SOHCAHTOA and the Pythagorean Theorem
The first thing you need to know basically puts the tri- in trig. It is all about using right triangles to find missing angle measures and side lengths. There are 3 basic functions that relate an angle measure (except the 90 degree angle) to two out of the three sides of a triangle: sine, cosine, and tangent.
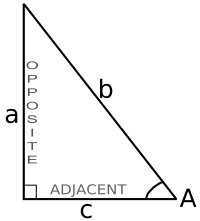
When we take the sine, cosine, or tangent of an angle, we are given a number expressed as a ratio of two sides. We use the Greek symbol theta to express an unknown angle measure as a variable. To easily remember which function relates to which two sides, we use the acronym SOHCAHTOA.
To get a detailed breakdown of this concept, take a look at these two videos:
SOHCAHTOA: part 1
SOHCAHTOA: part 2
Pythagorean theorem states that a^2 + b^2 = c^2, where a and b are the legs of the triangle and c is always the hypotenuse. The hypotenuse is the side opposite the 90 degree angle, or the longest side of the right triangle.
Three More Functions: Secant, Cosecant, and Cotangent
In addition to sine, cosine, and tangent, there are three more trig functions that you need to know. Since they are just reciprocal functions of what we just learned, they are quite simple to remember:
1/cos = secant (or sec)
1/sin = cosecant (or csc)
1/tan = cotangent (or cot)
Secant corresponds with cosine, cosecant corresponds with sine, and cotangent corresponds with tangent. Now, you just have to keep in mind that secant doesn’t go with sine and cosecant doesn’t go with cosine; it’s backwards. Other than that, it’s pretty simple.
Trigonometric Identities
There are some handy equations that are vital to helping you solve questions or reach the answer in a different way. Though you do not need to memorize all of them (since there are very many), you should know all of these by heart:
sine/cosine = tangent
sine^2 x + cos^2 x =1
tan^2 + 1 = sec^2 x
cot^2 + 1 = csc^2 x
Radians
Radians express angle measure as a ratio of the arc length to the radius. You already know pi, which the number of diameters it takes to go all the way around a circle. Since the radius is half of the diameter, 2pi radians are equal to 360 degrees.
Radians are another way to think of angle measures. Why do people use radians? It’s easier in some cases to use radians – the math is easier, it’s easier to think of 5pi radians than 900 degrees, etc.
360 degrees = 2pi radians.
How to convert from radians to degrees and vice versa:
Degrees = Radians times (180/pi)
Radians = Degrees times (pi/180)
In most problems, if you are looking at the unit circle or at a graph, then the angle measures will be in radians. If you are just looking at triangles, then the angle measures will usually be in degrees.
Remember to check if your calculator is in radians or degrees. Having your calculator on the wrong setting is a very common mistake, one that SAT test makers are sure to exploit, so watch out!
The Unit Circle
I know what you’re probably thinking “Wait, there’s more than just triangles?” Yes, you do have to know your unit circle in addition to your right triangles, but I promise that it’s not as painful as it sounds.
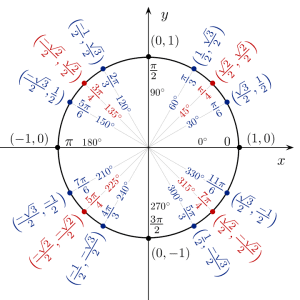
Photo by Jim.belk
The equation of the unit circle looks similar to the standard equation of a circle: x^2 + y^2 = 1.
One of the biggest limitations to the SOHCAHTOA triangle is that you are constrained to angles between 0 and 90 degrees. If you wanted to figure out the sine of 150 degrees, you can’t do that with basic right triangle trigonometry. You can’t even properly determine the sine of 0 or 90 degrees.
The unit circle solves that problem by extending the possibilities to beyond 90 degrees. The right triangle is still used, but it is now applied to the larger context of a circle.
In order to find the solution to trigonometric functions in quadrant 2, 3, or 4, we have to use something called a reference angle. This means taking what we already know from quadrant 1 (using SOHCAHTOA) and applying it to the rest of the picture.
Let’s say we wanted to figure out the sine of 5pi/6 (150 degrees). We first figure out which quadrant 5pi/6 appears in. In this case, it’s quadrant 2. Then we figure out how many degrees theta is away from the x-axis (in the same quadrant). Since 5pi/6 is equivalent to 150 degrees, it takes 30 more degrees to reach the x-axis in quadrant 2.
Now, all we have to do is take sin pi/6 (30 degrees) and use it as the reference angle. This is the easy part. The numerical value of sin 5pi/6 is the same as its reference angle, so the answer is ½. How do we figure out whether our answer is positive or negative?
Using the SOHCAHTOA triangle, we could only get positive answers from trig functions since it doesn’t make sense to have negative triangle side lengths. However, with the unit circle, it is possible to get a negative answer when we take the sine, cosine, and tangent of angles beyond the first quadrant.
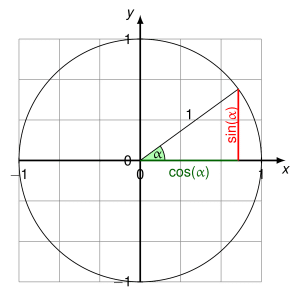
Photo by
Imagine a triangle drawn in the first quadrant with theta being the angle formed by the x-axis and a line extending all the way out to the unit circle. The sine of theta is opposite over hypotenuse, and the cosine of theta is adjacent over hypotenuse.
When we take the sine of a function, we get the y-coordinate of the point where the triangle hits the unit circle. When we take the cosine, we get the x-coordinate instead. It’s not necessary to know the reasoning behind this, but you can try comparing different values of sine and cosine, looking at the unit circle, and then thinking this one through for yourself.
Since sine corresponds with the y-coordinate, its function is positive in the first two quadrants. Cosine corresponds with the x-coordinate, and the function is positive in quadrant 1 and 4.
Tangent is sine divided by cosine, so it’s positive where both sine and cosine are the same sign. In other words, taking the tangent of an angle measure yields a positive answer if the angle resides in either quadrant 1 or 3.
Sine, cosine, and tangent are all defined at 0, pi/2, pi, and 3pi/2. If you don’t already know the solutions, go ahead and take a look at the graphs on the next section.
Graphing the Basic Trig Functions
You will need to be comfortable with graphing functions, matching graphs to equations, and understanding how to transform a graph. If you have a graphing calculator, make sure to follow along by punching in the equations. If you don’t have one yet, you can use Wolfram Alpha in the meantime.
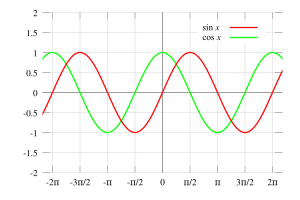
Photo by Qualc1
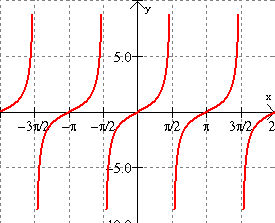
Photo by 4.bp.blogspot.com
sin(x) = y
cos(x) = y
tan(x) = y
x = radians or degrees
y = the function output
This is the standard equation of a standard graph for sine, cosine, and tangent. As you can see, the graphs repeat over and over. The tangent graph repeats every pi radians while sine and cosine repeat every 2pi radians.
The sine and cosine graphs go horizontally and tangent goes vertically. Sine and cosine never go beyond y =1 and –1. Can you explain why?
Hint: review what the sine, cosine, and tangent functions represent.
How do we get the graph of sine, cosine, and tangent? The answer can be found by looking at the unit circle. When we look at sin(x) = y, x is theta, and the y is equal to the y-coordinate at the point where the right triangle touches the unit circle.
As you can see, we can input any number for the angle measure, but our output is constrained by 1 and –1. For cos(x) = y, the y is equal to the x-coordinate at the point where the right triangle touches the unit circle.
To account for the shape of the sine and cosine graph:
In quadrant 1, y increases and x decreases as we go along the unit circle. In quadrant 2, as theta increases from pi/2 to pi, and both x and y decrease. In quadrant 3, x increases and y decreases. In quadrant 4, both x and y increase. Notice that in certain quadrants, x and y are either negative or positive. This is the reason for sine and cosine’s wavy shape.
sin(x) = sin(x + 2pi)
cos(x) = cos(x + 2pi)
Tangent’s graph looks a little funky, but if you understand that tan = sin/cos, then you will see why it looks like that.
The period is the horizontal distance required to draw one repetition of the curve. Since the standard unit circle is 2pi radians long, the standard period for the sin and cosine graph is 2pi. Tangent is a bit tricky, repeating every pi radians.
Challenge question: Can you see why the tangent graph repeats every pi radians and not every 2 pi radians?
Hint: think about the definition of tangent, then apply it to the unit circle starting from quadrant 1 going all the way around to quadrant 4.
(graph of secant, cosecant, and cotangent)
To find the graph of secant, cosecant, and cotangent, just take the reciprocal of the sine, cosine, and tangent functions. Plug them into your calculator, mess around with different values and variables, and see how they are similar and different from the basic three trig functions.
Transforming the Graph
Asin(Bx + C) + D = y
By changing the variables A, B, C, or D, you can make the graph bigger or smaller. You can even change the position of the graph or flip it upside-down. I used sine throughout the examples listed below, but the rules apply to cosine as well.
The A variable, which affects the amplitude, stretches the graph vertically. The peaks and valleys will extend farther out for numbers with an absolute value higher than 1. For numbers that have an absolute value between 0 and 1, the graph will contract vertically and will be smaller than y = 1 and –1. For example, .5sin(x) = y will make the maximum and minimum equal to y = .5 and – .5. If A is negative, then the graph becomes flipped over.
D moves the graph up or down by D units. The graph itself stays unchanged, but the position of the wave shifts. Imagine picking up the entire graph and laying it back down higher or lower lower than the original. For example, sin(x) + 5 = y moves the graph up by 5 units.
While A and D affect the graph vertically, B and C control the graph horizontally. B affects the period, or the length (in radians) it takes to draw one complete cycle. The higher the number, the more squished in the graph will look compared to the original version. Like A, if B is negative, the graph flips upside-down.
C moves the graph left or right. Like D, C only affects the position of the graph, not the shape. A negative number shifts the graph to the right and a positive number shifts the graph to the left.
Special Right Triangles
There are some common “special” triangles that you will see over and over again on the SAT, in school, and even in real life. You don’t necessarily have to know them off the top of your head, but it could potentially save you a lot of time, so it’s worth taking a look at.


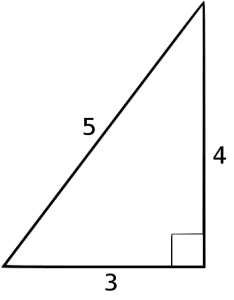
Start familiarizing yourself with them now so that as they come up during your study time, you can start to recognize them.
In addition to knowing the common angle measures and the corresponding side length ratios, it is also helpful to know the sine, cosine, and tangent values for pi/6, pi/4, and pi/3 along with the corresponding positions on the unit circle.
You can use the 30-60-90 triangle and the 45-45-90 triangle in quadrant 1 as reference angles to figure out what goes in quadrant 2, 3, and 4. All you have to do is add or subtract from pi, 3pi/2, or 2pi to get the angle measure for those quadrants.
Conclusion
If you haven’t touched on or learned trig in school yet, this can be overwhelming, especially because of the depth of knowledge required to fully understand the material.
Give yourself at least 4-6 weeks and break each section down into digestible chunks. Spend about 15-30 minutes each night studying a small section.
If you don’t have that much time left before the actual SAT exam, I recommend focusing on SOHCAHTOA and getting that grooved into your memory. Good luck and happy studying!






Leave a Reply