In this episode of TuesdACT, we are taking a look at the principles of multiplying and adding even and odd numbers using an example from The Real ACT Prep Guide that lots of students struggle with.
Real ACT Prep Guide Test 4 Question 48 on page 599, we are coming for you!
Check out the video or read below for the explanation and a little lesson on even and odd integers.
If 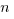 is a positive integer, which of the following expressions must be an odd integer?
is a positive integer, which of the following expressions must be an odd integer?
F. 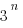
G. 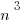
H. 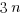
J. 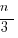
K. 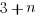
Alright, let’s pause and go over principles of adding, subtracting, and multiplying odd and even numbers. Here’s how it shakes out.
Even + Even = Even
Even + Odd = Odd
Odd + Odd = Even
Even x Even = Even
Even x Odd = Even
Odd x Odd = Odd
You don’t need to memorize the rules; if you forget, just go through the examples with easy numbers like 2 and 3 and see what happens. It will be the same for all even and odd numbers.
So, for example:
Even + Even = Even 2 + 2 = 4
Even + Odd = Odd 2 + 3 = 5
Odd + Odd = Even 3 + 3 = 6
Even x Even = Even 2 x 2 = 4
Even x Odd = Even 2 x 3 = 6
Odd x Odd = Odd 3 x 3 = 9
Question 48 is also a great example of how you can find important clues in the answer choices on the ACT. Notice that all of our answer choices have the number 3 in them, so all of our answer choices are doing something with an odd number.
Now let’s test with values for 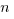 . Don’t forget that we don’t know whether
. Don’t forget that we don’t know whether 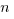 is even or odd, so we need to test for both cases.
is even or odd, so we need to test for both cases.
Answer Choice F:
3 is raised to a power of 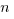 . If
. If 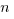 is even (2), this gives us 3 x 3 (ODD x ODD = ODD). If
is even (2), this gives us 3 x 3 (ODD x ODD = ODD). If 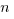 is odd (3), this gives us 3 x 3 x 3 (ODD x ODD x ODD), which is also always odd.
is odd (3), this gives us 3 x 3 x 3 (ODD x ODD x ODD), which is also always odd.
Since an odd number multiplied by an odd number is always an odd number, and because in this case we are just multiply 3 (an odd number) by itself a number of times, it will always be odd. So F is our answer, but let’s check the rest.
Answer Choice G:
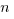 is raised to a power of 3. So if
is raised to a power of 3. So if 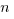 is odd, then we have 3 x 3 x 3 (ODD x ODD x ODD) and we know that ODD x ODD = ODD. But if
is odd, then we have 3 x 3 x 3 (ODD x ODD x ODD) and we know that ODD x ODD = ODD. But if 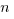 is even then we have 2 x 2 x 2, and EVEN x EVEN = EVEN. So
is even then we have 2 x 2 x 2, and EVEN x EVEN = EVEN. So 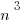 is not necessarily odd.
is not necessarily odd.
Answer Choice H:
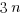 . So if
. So if 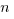 is even (2), we have 3 x 2 (ODD x EVEN = EVEN); if
is even (2), we have 3 x 2 (ODD x EVEN = EVEN); if 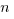 is odd, we have 3 x 3 (ODD x ODD = ODD). So
is odd, we have 3 x 3 (ODD x ODD = ODD). So 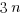 is not necessarily odd.
is not necessarily odd.
Answer Choice J:
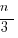 . This is an interesting one, because if we pick a value for
. This is an interesting one, because if we pick a value for 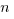 that does not divide evenly by 3, then it is actually neither even nor odd. By rule, the terms even or odd apply only to integers not to fractions.
that does not divide evenly by 3, then it is actually neither even nor odd. By rule, the terms even or odd apply only to integers not to fractions.
Answer Choice K:
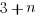 . So if
. So if 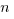 is even (2), then we have 3 + 2 (ODD + EVEN = ODD); if
is even (2), then we have 3 + 2 (ODD + EVEN = ODD); if 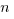 is odd, then we have 3 + 3 (ODD + ODD = EVEN). So
is odd, then we have 3 + 3 (ODD + ODD = EVEN). So 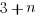 is not necessarily odd.
is not necessarily odd.
So our answer is F.






Leave a Reply