Few topics perplex students like circles on the GMAT. “What was the formula for area of a circle, again? I know pi is in there somewhere!” With a few simple insights, though, this entire topic simplifies. First, let’s look at a problem. How do you find the do calculations for something like this?
Given that O is the center of the circle, and given the info in the diagram, how do we find (1) the length of arc AB? , and (2) the area of sector AOB (i.e. the shaded region)? BTW, the word sector is the name for a “slice of pie” piece of a circle, and arclength is the curved length along the “crust” of the pie. You can find a little more about arclength at this post.
Proportions
One of the greatest math “tricks” is proportional reasoning, and this is precisely what unlocks all the questions posed above. Basically, to find either the length of an arc or the area of sector, we set up a part-to-whole ratio, and set this equal to a ratio for the angle. In the diagram, the angle at the center of the circle is 80°. A whole circle, all the way around, is always 360°, so this 80° angle takes up 80/360 = 8/36 = 2/9 of a circle. Therefore, both the arclength and the area of the sector are 2/9 of their respective “wholes”.
Arclength
If arclength is the “part”, then the “whole” is the length all the way around the circle — we call that length circumference, and its formula is
The part-over-whole of the angles has to equal the part-over-whole of these lengths:
For this diagram, this proportion becomes:
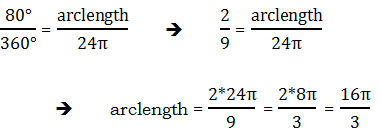
Area of a sector
If area of the sector is the “part”, then the area of the whole circle is the whole. Of course, for the area of a circle, we use Archimedes‘ remarkable formula:
As with arclength, the part-over-whole of the angles has to equal the part-over-whole of these areas:
For this diagram, this proportion becomes:
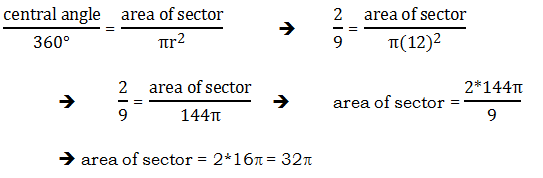
BTW, a mental math tip — how did I just know, in my head, that 144/9 = 16, or in other words, that 9*16 = 144? Well, think about it.
That logic makes it very easy to find factors of perfect squares.
Summary
If you remember these simple proportional reasoning tricks, you will be able to figure out anything the GMAT might ask about a part of a circle. Here’s a free practice question on which to apply these skills.
1) http://gmat.magoosh.com/questions/87
This article is the fifth in a series of five articles on circles. Here’s the rest of the series:
1) Introduction to Circles on the GMAT
2) GMAT Geometry: Circles and Angles
3) Circle and Line diagrams on the GMAT
4) Inscribed and Circumscribed Circles and Polygons on the GMAT
5) Slicing up GMAT Circles: Arclengths, Sectors, and Pi



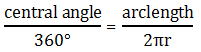
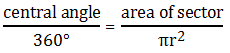



Leave a Reply